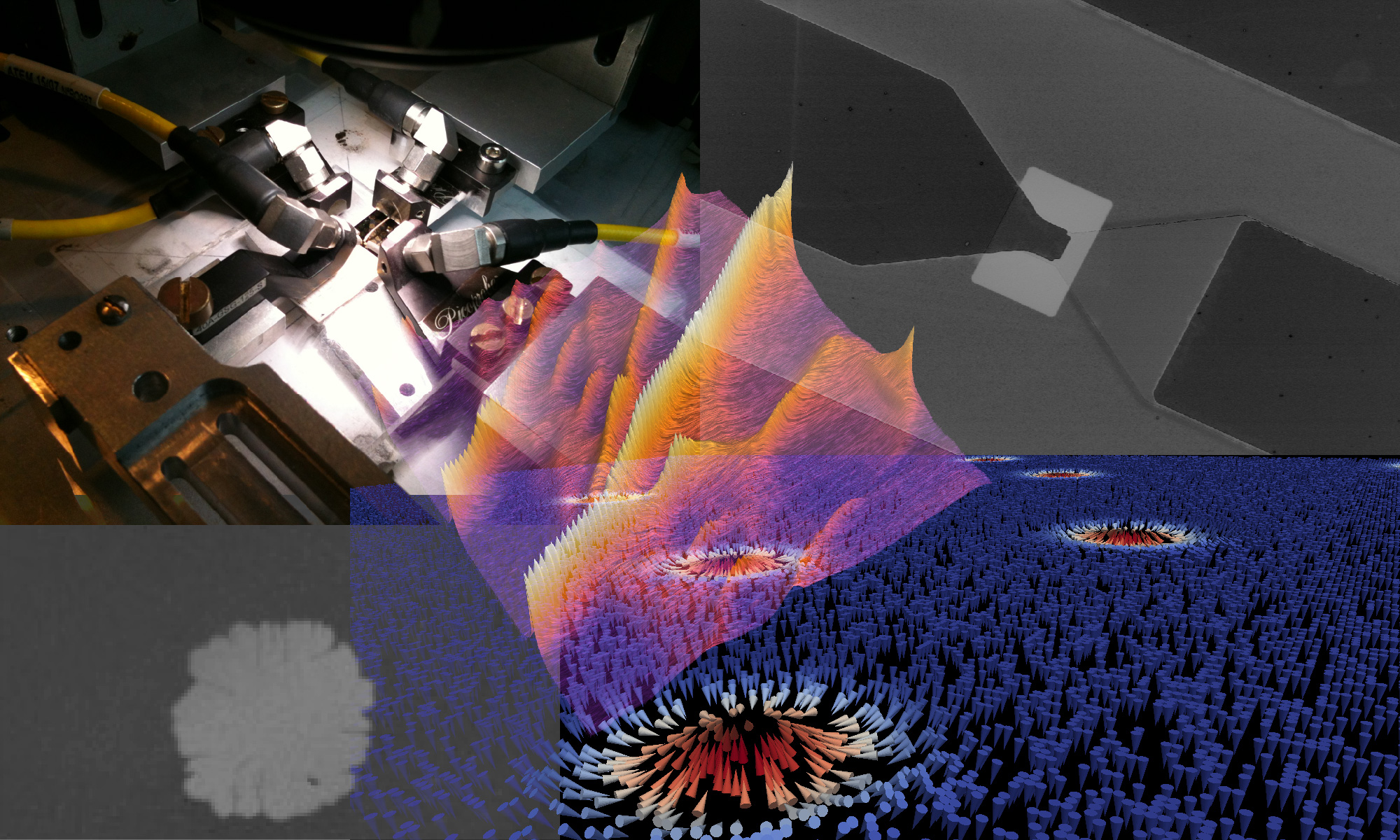
Scientific project
Ferromagnets are ordered spin systems in which a variety of nonlinear phenomena are possible. An interesting example in thin films is the magnetic vortex, which comprises a topological spin structure in which moments circulate in the plane of the film but culminate in a perpendicular direction at the vortex core – a compact region 10-20 nm in size. When an electrical current is applied to the vortex the flow of electron spins exert a torque on the magnetic moments, which leads to novel dynamics like steady state gyration of the vortex core that is inherently non-Newtonian [1].
In this project, we will explore strongly nonlinear phenomena such as the reversal of the vortex core magnetization, which occurs when a threshold in the core dynamics is reached. When this reversal is periodic a new time scale is introduced, which competes with the gyration frequency and leads to commensurate and incommensurate states that can have a fractal character like “Devil’s Staircases” [2]. Moreover, these incommensurate states and suspected to be chaotic, a rare phenomenon in spintronic devices that can open up new possibilities for information processing [3]. However, the physical mechanism leading to core reversal on the nanoscale remains an open question and a challenging problem is to construct a physical theory that allows the routes to chaos to be identified and understood.
This work will be carried out in collaboration with research groups at the Unité Mixte de Physique CNRS/Thales (Palaiseau), Institut Jean Lamour (Nancy), and CentraleSupélec (Metz).
References
[1] Q. Mistral et al., Physical Review Letters, vol. 100, no. 257201 (2008).
[2] S. Petit-Watelot et al., Nature Physics, vol. 8, pp. 682-687 (2012).
[3] M. Sciamanna and K. Shore, Nature Photonics, vol. 9, pp. 151-162 (2015).
Methods and techniques
The research will comprise experimental and theoretical work. In experiment, high-frequency electrical device characterization will be carried out at cryogenic temperatures. The theoretical techniques used will include the theory of nonlinear dynamical systems, micromagnetics simulations, theory of stochastic processes, and basic programming.
Supervisors
Thibaut Devolder, Joo-Von Kim
Start date and duration
This project can start in October 2016 with a duration of three years.